Rigidity theory aims to determine whether a set of pairwise distances (represented as edges) between a collection of points (represented as vertices) uniquely determines their position up to rigid transformations (rotations, translations and reflexions). For example, the object in the left is rigid, while the one in the right (known as the double banana) is not.

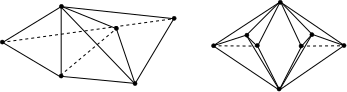
Rigidity has a tight relation with subspaces and missing data, as one only observes a subset of all the distances, and distance matrices are low-rank (all its columns lie in a low-dimensional subspace).

Rigidity is a fundamentalproblem with applications in fields as diverse as chemistry, echolocation and graph theory. However, a characterization of rigid structures remains an open question since the times of Lagrange and Maxwell. Our theory for missing data might help expand our knowledge of rigidity.